Force, Motion and Gravitation

Center of mass

The center of mass is the average distance, weighted by mass

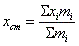
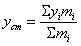
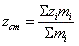
In a Cartesian coordinate, the center of mass is the point obtained by doing a weighted average for all the positions by their respective masses.
The center of mass of the Earth and a chicken in space is going to be almost at the center of the Earth, because the chicken is tiny, and its coordinate is weighted so.
The center of mass between two chickens in space is going to be right in the middle of the two chickens, because their positions are weighted equally.
You do not have to obtain the absolute coordinates when calculating the center of mass. You can set the point of reference anywhere and use relative coordinates.
The center of mass for a sphere is at the center of the sphere.
The center of mass of a donut is at the center of the donut (the hole).

Newton's first law, inertia

The law of inertia basically states the following: without an external force acting on an object, nothing will change about that object in terms of speed and direction.

In the absence of an external force:

Something at rest will remain at rest
Something in motion will remain in motion with the same speed and direction.
Objects are "inert" to changes in speed and direction.

Newton's second law (F = ma)

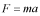
A net force acting on an object will cause that object to accelerate in the direction of the net force.

The unit for force is the Newton. N = ^kg·m/_s²
Both force and acceleration are vectors because they have a direction.
Many MCAT questions omit the direction attribute because it is so obvious. For example, when an apple falls to the ground (or on Newton), we all know that the force of gravity acts downwards, and the apple of course, falls downwards. Questions in this scenario are just simple cases of plugging in the formula
However, more difficult questions have directional attributes associated with them. For example, when a bar of soap slides down an inclined plane, the force of gravity acts downwards, but the acceleration is not completely downwards, but is "slanted". Therefore, you need to do vector analysis (simple ones only. The MCAT is too short for complex, time-consuming ones that appear in your physics midterm).

Newton's third law, forces equal and opposite

Every action has an equal and opposite reaction

for the MCAT, you need to know that this law applies to propulsion. This is why rockets work even in the vacuum of space.

Concept of a field

For the purposes of the MCAT, fields are lines.
When lines are close together, that's shows a strong field.
When lines are far apart, that shows a weak field.
Lines / fields have direction too, and that means they are vectors.
Things travel parallel, perpendicular, or spiral to the field line.

Law of gravitation (F = Gm1m2/r^2)

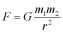
Gravity decreases with the square of the distance.
If the distance increases two fold, gravity decreases by a factor of four.
The "distance" is the distance from the center of mass between the two objects.
Gravity is the weakest of the four universal forces.
This weakness is reflected in the universal gravitational constant, G, which is orders of magnitude smaller than the Coulomb's constant.

Uniform circular motion

Memorize the equations

acceleration:
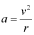
force:
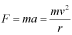
circumference:
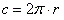
arc:
area:
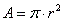
sector:
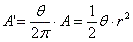
note that theta is always in radians. 2pi radians = 360 degrees.
The simple harmonic laws of frequency and period applies here also.

Get the concepts

Distinguish between velocity and speed: Velocity is displacement over time. Speed is the distance over time.
Displacement is the shortest, straight-line distance between two points on the perimeter of a circle (technically, this is called the chord). Distance is circumference and arc.
Some typical cases:
- For displacements and distances that approach zero, the instantaneous velocity equals the speed.
- For a quarter around the circle (pi/2 radians or 90 degrees), the displacement is the hypotenuse of a right-angled triangle with the radius as the other two sides. Using Pythagoras, the displacement is square root of 2r^2. The distance is the arc of 1/4 circumference.
- For half around the circle, the displacement is the diameter and the distance is half the circumference.
- For three quarters around the circle, the displacement is again obtained by Pythagoras. The magnitude of the displacement here is the same as that at a quarter of a circle, but the direction is different. The distance, is 3/4 of the circumference.
- Complete around the circle, the displacement is zero, which makes the velocity also zero. The distance is the circumference.
The velocity is always less or equal to the speed.
The displacement is always less or equal to the distance.
Displacement and velocity are vectors. Distance and speed are not.
Moving around a circle at constant speed is also simple harmonic motion.
frequency = how many times the object goes around the circle in one second.
period = time it takes to move around the entire circle.

Centripetal Force (F=-mv²/r)

Centripetal force is due to centripetal acceleration. Centripetal acceleration is due to changes in velocity when going around a circle. The change in velocity is due to a constant change in direction.

Centripetal force:
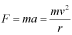
- Sometimes a negative sign is used for centripetal force to indicate that the direction of the force is toward the center of circle.
Centripetal acceleration:
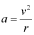
The direction of both the acceleration and the force is toward the center of the circle.
The tension force in the string (attached to the object going in circles) is the same as the centripetal force.
When the centripetal force is taken away (Such as when the string snaps), the object will fly off in a path tangent to the circle at the point of snap.

Weight

Weight is the force that acts on a mass

Weight is a force. It has a magnitude and a direction. It is a vector.
Because it is a force, F=ma holds true.
Your weight on the surface of the Earth: F=mg, where g is the acceleration due to Earth, which is just under 10.
You weigh more on an elevator accelerating up because F=mg + ma, where a is the acceleration of the elevator.
An elevator accelerating up is the same thing as an elevator decelerating on its way down, in terms of the acceleration in F=mg + ma.
You weigh less on an elevator accelerating down because F=mg - ma, where a is the acceleration of the elevator.
An elevator accelerating down is the same thing as an elevator decelerating on its way up, in terms of the acceleration in F=mg - ma.
You weigh less when you are further away from the Earth because the force of gravity decreases with distance.
However, you are not truly "weightless" when orbiting the Earth in space. You are simply falling toward the Earth at the same rate as your space craft.
You gain weight as you fall from space to the surface of the earth.
For a given mass, its weight on Earth is different from its weight on the Moon.
When something is laying still on a horizontal surface, the normal force is equal and opposite to the weight.
When something is laying still on an inclined plane, the normal force and friction force adds up in a vector fashion to equal the weight.

Friction, static and kinetic

Friction is a force that is always in the direction to impede the sliding of surfaces.

Static friction: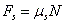

Kinetic friction: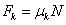

u is the coefficient of friction and N is the normal force.

Like any other force, friction is a vector. However, its direction is easy because it's always opposite to the motion of the surface involved.
Static friction pertains to objects sitting still. An object can sit still on an inclined plane because of static friction.
Kinetic friction pertains to objects in motion. A key sliding across the table eventually comes to a stop because of kinetic friction.
Static friction is always larger than kinetic friction.
The coefficient static friction is always larger than the coefficient of kinetic friction.
The coefficient of friction is intrinsic to the material properties of the surface and the object, and is determined empirically.
The normal force at a horizontal surface is equal to the weight
The normal force at an inclined plane is equal to the weight times the cosine of the incline angle (see inclined planes).
We can walk and cars can run because of friction.
Lubricants reduce friction because they change surface properties and reduce the coefficient of friction.
Every time there is friction, heat is produced as a by-product.

Motion on an inclined plane

Gravity is divided into two components on an inclined plane.
- One component is normal (perpendicular) to the plane surface: F_N = mg·cosθ
- The other component is parallel to the plane surface: F_|| = mg·sinθ
To prevent the object from crashing through the surface of the inclined plane, the surface provides a normal force that is equal and opposite to the normal component of gravity.
Friction acts parallel to the plane surface and opposite to the direction of motion.
In a non-moving object on an inclined plane: normal component of gravity = normal force; parallel component of gravity = static friction.
Unless the object levitates or crashes through the inclined plane, the normal force always equals the normal component of gravity.
In an object going down the inclined plane at constant velocity: parallel component of gravity = kinetic friction (yes, they're equal, don't make the mistake of thinking it's larger. Constant velocity = no acceleration = no net force).
In an object that begins to slip on the inclined plane: parallel component of gravity > static friction.
In an object that accelerates down the inclined plane: parallel component of gravity > kinetic friction.
When you push an object up an inclined plane, you need to overcome both the parallel component of gravity and friction.
When you push or pull an object up an inclined plane, make sure you divide that force into its components. Only the component parallel to the plane contributes to the motion.

Analysis of pulley systems

Pulleys reduce the force you need to lift an object. The catch - it increases the required pulling distance.

For the purpose of the MCAT, just memorize the simple pulley systems below.
Rule of thumb: The ropes on either side of a moving pulley contributes to pulling the load.
The MCAT will most probably give you simple pulleys where only the above rule is applicable.
Complex pulleys will have additional ropes that contribute to the pulling of the load (most likely not tested on the MCAT).
The distance of pulling increases by the same factor that the effort decreases.

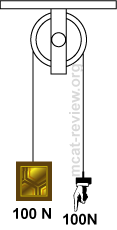
There are no moving pulleys here. If the weight of the box is 100 N, you have to pull with a force of 100 N. For every 1 meter you pull, the box goes up 1 meter.

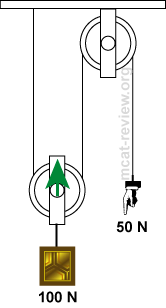
When there is one moving pulley, the force needed to pull is halved because strings on both side of the pulley contribute equally. You supply 50 N (which is transmitted to the right-hand rope) while the left-hand rope contributes the other 50 N. Because effort here is halved, the distance required to pull the box is doubled.

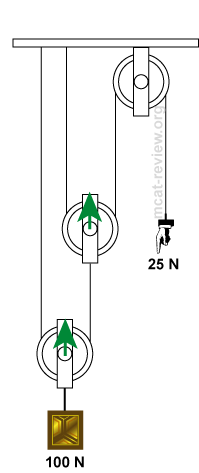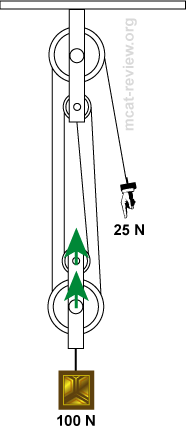
There are two moving pulleys here. Counting the ropes reveal that when we tug on one rope, it gets transmitted to a system where 4 ropes pull on the load. Thus, you can pull the 100 N box with only 25 N. However, for every 4 m you pull, the box only goes up 1 m.

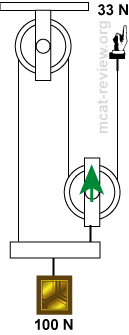
This is a complex pulley. Just like the simple pulleys, the ropes on both sides of the moving pulley contribute. Here, the left-most rope contributes also. This makes 3 contributing ropes, which makes the effort required to be reduced by a factor of 3. The distance you need to pull here is 3 times the distance the box will travel.

Force

There are 4 universal four-ces... get it?
Universal forces are also called fundamental forces.
The four forces are:
- The strong force: also called the nuclear force. It is the strongest of all four forces, but it only acts at subatomic distances. It binds nucleons together.
- Electromagnetic force: about one order of magnitude weaker than the strong force, but it can act at observable distances. Binds atoms together. Allows magnets to stick to your refrigerators. It is responsible for the fact that you are not falling through your chair right now (MCAT people love to throw you quirky examples like this one).
- Weak force: roughly 10 orders of magnitude weaker than the strong force. Responsible for radioactive decay.
- Gravity: roughly 50 orders of magnitude weaker than the strong force. Responsible for weight (not mass!). Also, responsible for planet orbits.